Dams and Different parts & terminologies of Dams ?

Dams

A dam is a hydraulic structure of fairly impervious material built across a river to create a reservoir on its upstream side for impounding water for various purposes. These purposes may be Irrigation, Hydropower, Water-supply, Flood Control, Navigation, Fishing, and Recreation. Dams may be built to meet the one of the above purposes or they may be constructed fulfilling more than one. As such, Dam can be classified as Single-purpose and Multipurpose Dam.

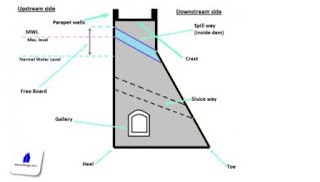
Different parts & terminologies of Dams:

Crest: The top of the Dam. These may in some cases be used for providing a roadway or walkway over the dam.

Dam illustration

Parapet walls: Low Protective walls on either side of the roadway or walkway on the crest.

Heel: Portion of Dam in contact with ground or river-bed at upstream side.

Toe: Portion of dam in contact with ground or river-bed at downstream side.

Spillway: It is the arrangement made (kind of passage) near the top of dam for the passage of

surplus/ excessive water from the reservoir.

Abutments: The valley slopes on either side of the dam wall to which the left & right end of dam

are fixed to.

Gallery: Level or gently sloping tunnel like passage (small room like space) at transverse or

longitudinal within the dam with drain on floor for seepage water. These are generally provided

for having space for drilling grout holes and drainage holes. These may also be used to

accommodate the instrumentation for studying the performance of dam.

Sluice way: Opening in the dam near the base, provided to clear the silt accumulation in the

reservoir.

Free board: The space between the highest level of water in the reservoir and the top of the dam.

Dead Storage level: Level of permanent storage below which the water will not be withdrawn.

Diversion Tunnel: Tunnel constructed to divert or change the direction of water to bypass the dam

construction site. The dam is built while the river flows through the diversion tunnel.

Various types of dams

Dams can be classified in number of ways. But most usual ways of classification of dams are mentioned below:

Based on the functions of dam, it can be classified as follows:

Storage dams: They are constructed to store water during the rainy season when there is a large flow in

the river. Many small dams impound the spring runoff for later use in dry summers. Storage dams may also provide a water supply or improved habitat for fish and wildlife. They may store water for hydroelectric power generation, irrigation, or for a flood control projects. Storage dams are the most common type of dams and in general, the dam means a storage dam unless qualified otherwise.

Diversion dams: A diversion dam is constructed for the purpose of diverting water from the river into an offtaking canal (or a conduit). They provide sufficient pressure for pushing water into ditches, canals, or other conveyance systems. Such shorter dams are used for irrigation, and for diversion from a stream to a distant storage reservoir. A diversion dam is usually of low height and has a small storage reservoir on its upstream. The diversion dam is a sort of storage weir which also diverts water and has a small storage. Sometimes, the terms weirs and diversion dams are used synonymously.

Detention dams: Detention dams are constructed for flood control. A detention dam retards the flow in the river on its downstream during floods by storing some flood water. Thus the effect of sudden floods is reduced to some extent. The water retained in the reservoir is later released gradually at a controlled rate according to the carrying capacity of the channel downstream of the detention dam. Thus the area

downstream of the dam is protected against flood. 

Debris dams: A debris dam is constructed to retain debris such as sand, gravel, and drift wood flowing in the river with water. The water after passing over a debris dam is relatively clear. 

Coffer dams: It is an enclosure constructed around the construction site to exclude water so that the construction can be done in dry. A cofferdam is thus a temporary dam constructed for facilitating

construction. A coffer dam is usually constructed on the upstream of the main dam to divert water into a

diversion tunnel (or channel) during the construction of the dam. When the flow in the river during

construction of the dam is not much, the site is usually enclosed by the coffer dam and pumped dry.

Sometimes a coffer dam on the downstream of the dam is also required.

Based on structure and design, dams can be classified as follows:

Gravity Dams: A gravity dam is a massive sized dam fabricated from concrete or stone masonry. They are designed to hold back large volumes of water. By using concrete, the weight of the dam is actually able to resist the horizontal thrust of water pushing against it. This is why it is called a gravity dam. Gravity essentially holds the dam down to the ground, stopping water from toppling it over.

Gravity dams are well suited for blocking rivers in wide valleys or narrow gorge ways. Since gravity dams must rely on their own weight to hold back water, it is necessary that they are built on a solid foundation of bedrock.

Examples of Gravity dam: Grand Coulee Dam (USA), ( Nagarjuna Sagar Dam (India) and Itaipu Dam

( Between Brazil and Paraguay).

Earth Dams: An earth dam is made of earth (or soil) built up by compacting successive layers of earth,

using the most impervious materials to form a core and placing more permeable substances on the

upstream and downstream sides. A facing of crushed stone prevents erosion by wind or rain, and an ample spillway, usually of concrete, protects against catastrophic washout should the water overtop the dam.Earth dam resists the forces exerted upon it mainly due to shear strength of the soil. Although the weight ofthe earth dam also helps in resisting the forces, the structural behavior of an earth dam is entirely different from that of a gravity dam. The earth dams are usually built in wide valleys having flat slopes at flanks (abutments). The foundation requirements are less stringent than those of gravity dams, and hence they can be built at the sites where the foundations are less strong. They can be built on all types of foundations.However, the height of the dam will depend upon the strength of the foundation material.

Examples of earthfill dam: Rongunsky dam (Russia) and New Cornelia Dam (USA).

Rockfill Dams: A rockfill dam is built of rock fragments and boulders of large size. An impervious

membrane is placed on the rockfill on the upstream side to reduce the seepage through the dam. The

membrane is usually made of cement concrete or asphaltic concrete. In early rockfill dams, steel and

timber membrane were also used, but now they are obsolete.

Mohale

Mohale dam, Lesoto Africa.

A dry rubble cushion is placed between the rockfill and the membrane for the distribution of water load

and for providing support to the membrane. Sometimes, the rockfill dams have an impervious earth core in the middle to check the seepage instead of an impervious upstream membrane. The earth's core is placed against a dumped rockfill. It is necessary to provide adequate filters between the earth core and the rockfill on the upstream and downstream sides of the core so that the soil particles are not carried by water and piping does not occur. The side slopes of rockfill are usually kept equal to the angle of repose of rock, which is usually taken as 1.4:1 (or 1.3:1). Rockfill dams require foundation stronger than those for earth dams.

Examples of rockfill dam: Mica Dam (Canada) and Chicoasen Dam (Mexico)

Arch Dams:  An arch dam is curved in plan, with its convexity towards the upstream side. An arch dam

transfers the water pressure and other forces mainly to the abutments by arch action. An arch dam is quite suitable for narrow canyons with strong flanks which are capable of resisting the thrust produced by the arch action.

Hoover Dam, USA

The section of an arch dam is approximately triangular like a gravity dam but the section is comparatively thinner. The arch dam may have a single curvature or double curvature in the vertical plane. Generally, the arch dams of double curvature are more economical and are used in practice.

Examples of Arch dam: Hoover Dam (USA) and Idukki Dam (India)

Buttress Dams: Buttress dams are of three types : (i) Deck type, (ii) Multiple-arch type, and (iii) Massivehead type. A deck type buttress dam consists of a sloping deck supported by buttresses. Buttresses are triangular concrete walls which transmit the water pressure from the deck slab to the foundation. Buttresses are compression members. Buttresses are typically spaced across the dam site every 6 to 30 metre, depending upon the size and design of the dam. Buttress dams are sometimes called hollow dams because the buttresses do not form a solid wall stretching across a river valley.The deck is usually a reinforced concrete slab supported between the buttresses, which are usually equally spaced.

buttress dam

In a multiple-arch type buttress dam, the deck slab is replaced by horizontal arches supported by buttresses. The arches are usually of small span and made of concrete. In a massive-head type buttress dam, there is no deck slab. Instead of the deck, the upstream edges of the buttresses are flared to form massive heads which span the distance between the buttresses. The buttress dams require less concrete than gravity dams. But they are not necessarily cheaper than the gravity dams because of extra cost of form work, reinforcement and more skilled labor. The foundation requirements of a buttress dam are usually less stringent than those in a gravity dam.

Examples of Buttress Dam: Bartlett dam (USA) and The Daniel-Johnson Dam (Canada)

Steel Dams: A steel dam consists of a steel framework, with a steel skin plate on its upstream face. Steel

dams are generally of two types: (i) Direct-strutted steel dams, and (ii)

steel dam

Cantilever type steel dams. In a direct strutted steel dam, the water pressure is transmitted directly to the

foundation through inclined struts. In a cantilever type steel dam, there is a bent supporting the upper part

of the deck, which is formed into a cantilever truss. This arrangement introduces a tensile force in the deck

girder which can be taken care of by anchoring it into the foundation at the upstream toe. Hovey suggested

that tension at the upstream toe may be reduced by flattening the slopes of the lower struts in the bent.

However, it would require heavier sections for struts. Another alternative to reduce tension is to frame

together the entire bent rigidly so that the moment due to the weight of the water on the lower part of the deck is utilized to offset the moment induced in the cantilever. This arrangement would, however, require bracing and this will increase the cost. These are quite costly and are subject to corrosion. These dams are almost obsolete. Steel dams are sometimes used as temporary coffer dams during the construction of the permanent dams. Steel coffer dams are supplemented with timber or earthfill on the inner side to make them water-tight. The area between the coffer dams is dewatered so that the construction may be done in dry for the permanent dam.

Examples of Steel Dam: Redridge Steel Dam (USA) and Ashfork-Bainbridge Steel Dam (USA)

Timber Dams: Main load-carrying structural elements of timber dam are made of wood, primarily

coniferous varieties such as pine and fir. Timber dams are made for small heads (2-4 m or, rarely, 4-8 m) and usually have sluices; according to the design of the apron, they are divided into piles, cribs, pile-crib, and buttressed dams.

Timber dam

The openings of timber dams are restricted by abutments; where the sluice is very long it is divided into

several openings by intermediate supports: piers, buttresses, and posts. The openings are covered by

wooden shields, usually several in row one above the other. Simple hoists—permanent or mobile

winches—are used to raise and lower the shields.

Forces Acting On Gravity Dam

In the design of a dam, the first step is the determination of various

forces that act on the structure and study their nature. Depending

upon the situation, the dam is subjected to the following forces:

1. Water pressure

2. Earthquake forces

3. Silt pressure

4. Wave pressure

5. Ice pressure

6. Self-weight of the dam.

The forces are considered to act per unit length of the dam.

For perfect and most accurate design, the effect of all the forces should

be investigated. Out of these forces, the most common and important forces

are water pressure and self-weight of the dam.

1. Water Pressure

Water pressure may be subdivided into the following two categories:

I) External water pressure:

It is the pressure of water on the upstream face of the dam. In this, there

are two cases:

(i) Upstream face of the dam is vertical and there is no water on the

downstream side of the dam

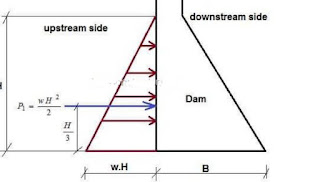Upstream face of the dam is vertical and there is no water on the downstream side of the dam

Figure 1

The total pressure is in horizontal direction and acts on the upstream face

at a height H/3 from the bottom. The pressure diagram is triangular and

the total pressure is given by P1=wH^2/2

Where w is the specific weight of water. Usually it is taken as unity.

H is the height upto which water is stored in m.

(ii) Upstream face with batter and there is no water on the downstream

side (figure 2).

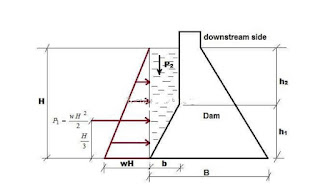downstream side

Here in addition to the horizontal water pressure P2 as in the previous

case, there is vertical P2 pressure of the water. It is due to the water column

resting on the upstream sloping side.

The vertical pressure acts on the length ‘b’ portion of the base. This

vertical pressure is given by P2=(bxh2xw)(1/2bxh1xw)

Pressure P2 acts through the centre of gravity of the water column resting

on the sloping upstream face.

If there is water standing on the downstream side of the dam, pressure

may be calculated similarly. The water pressure on the downstream face

actually stabilizes the dam. Hence as an additional factor of safety, it may

be neglected.Here in addition to the horizontal water pressure P2 as in the previous

case, there is vertical P2 pressure of the water. It is due to the water column

resting on the upstream sloping side.

The vertical pressure acts on the length ‘b’ portion of the base. This

vertical pressure is given by P2=(bxh2xw)(1/2bxh1xw)

Pressure P2 acts through the centre of gravity of the water column resting

on the sloping upstream face.

If there is water standing on the downstream side of the dam, pressure

may be calculated similarly. The water pressure on the downstream face

actually stabilizes the dam. Hence as an additional factor of safety, it may

be neglected.

II) Water pressure below the base of the dam or Uplift pressure

enters the pores and fissures of the foundation material under

pressure. It also enters the joint between the dam and the foundation at

the base and the pores of the dam itself. This water then seeps through

and tries to emerge out on the downstream end. The seeping water

creates a hydraulic gradient between the upstream and downstream side of

the dam. This hydraulic gradient causes vertical upward pressure. The

upward pressure is known as uplift. Uplift reduces the effective weight of

the structure and consequently the restoring force is reduced. It is

essential to study the nature of uplift and also some methods will have to

be devised to reduce the uplift pressure value.

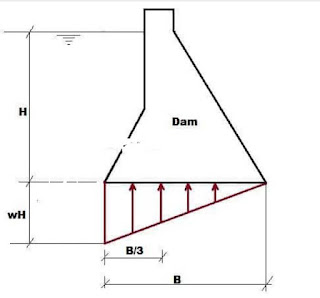Dam

Figure 3

With reference to figure 3, uplift pressure is given by Pu=(wHxB)/2

Where Pu is the uplift pressure, B is the base width of the dam, and H is

the height upto which water is stored.

This total uplift acts B/3 from the heel or upstream end of the dam.

Uplift is generally reduced by providing drainage pipes or holes in the dam

section.

The self-weight of the dam is the only largest force which stabilizes the

structure. The total weight of the dam is supposed to act through the

centre of gravity of the dam section in vertically downward direction.

Naturally when specific weight of the material of construction is high,

restoring force will be more. Construction material is so chosen that the

density of the material is about 2.045 gram per cubic meter

2. Earthquake Forces

The effect of an earthquake is equivalent to an acceleration to the foundation

of the dam in the direction in which the wave is travelling at the moment.

Earthquake wave may move in any direction and for design purposes, it is

resolved into the vertical and horizontal directions. On an average, a

value of 0.1 to 0.15g (where g = acceleration due to gravity) is generally

sufficient for high dams in seismic zones. In extremely seismic regions

and in conservative designs, even a value of 0.3g may sometimes by

adopted.

Vertical acceleration reduces the unit weight of the dam material and that

of water is to (1-Kv) times the original unit weight, where Kv is the value

of g accounted against earthquake forces, i.e. 0.1 when 0.1g is accounted

for earthquake forces. The horizontal acceleration acting towards the

reservoir causes a momentary increase in water pressure and the

foundation and dam accelerate towards the reservoir and the water

resists the movement owing to its inertia. The extra pressure exerted by

this process is known as hydrodynamic pressure.

3. Silt Pressure

If h is the height of silt deposited, then the forces exerted by this silt in

addition to the external water pressure, can be represented by Rankine

formula Psilt= 1/2𝛾s KaH2 acting at h/3 from the base.

Where,

Ka= coefficient of active earth pressure of silt = (1 - sinf)/(1 + sinf) 

f= angle of internal friction of soil, cohesion neglected.

𝛾s= submerged unit weight of silt material.

h = height of silt deposited.

Gravity Dams Silt Pressure • It has been explained under „ Reservoir Sedimentation‟ that silt gets deposited against the upstream face of the dam. If h is the height of silt deposited, then the force exerted by this silt in addition to external water pressure, can be represented by Rankine‟s formula as: • Psilt = ½ γ subw h2 Ka and it acts at h/3 from base • Where, Ka Is The Coefficient Of Active Earth Pressure of silt • Ka = 1 - sin Ө • 1+ sin Ө • Where Ө is the angle of internal friction of Soil, and cohesion is neglected. • γ subw = Submerged unit weight of silt material • h= height of silt deposited.

4.Wave Pressure

Wave Pressure • Waves are generated on the surface of the reservoir by the blowing winds, which causes a pressure towards the downstream side. Wave pressure depends upon the wave height. Wave height may be given by the equation • Hw= 0.032 √ V.F + 0.763 – 0.271 (F) ¾ for F < 32 Km And • Hw= 0.032 √V.F for F > 32 Km • Where hw= height of water from top of crest and bottom of trough in metre. • V= Wind velocity in Km/ hr

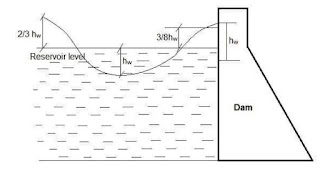Wave Pressure

5. Ice Pressure

The ice which may be formed on the water surface of the reservoir in cold

countries may sometimes melt and expand. The dam face is subjected to

the thrust and exerted by the expanding ice. This force acts linearly along

the length of the dam and at the reservoir level. The magnitude of this

force varies from 250 to 1500 kN/sq.m depending upon the temperature

variations. On an average, a value of 500 kN/sq.m may be taken under

ordinary circumstances.

6. Weight of dam

The weight of dam and its foundation is a major resisting force. In two

dimensional analysis of dam, unit length is considered

READ MORE

Dams and Different parts & terminologies of Dams?
Divide wall and main function
The layout of a Diversion Head Works and its components

Diversion Head Works and their objective? Weir and Barrage their types and difference?

What is Canal Fall?The necessity of Canal Falls and Types of Canal Falls

What are cross drainage works? Types of Cross-Drainage Works

Spillway and Necessity of Spillways