Moment of Resistance | Design of Singly reinforced Sections
Moment of Resistance | Guide to design of Singly reinforced Sections
For the design of Singly reinforced Sections article series, we have covered the following:
- Basic definitions and formulas
- Understanding stresses and modular ratios
- Assumptions for singly reinforced sections
- Design procedure for Singly reinforced section – I
- Solved Numericals for Singly reinforced beam | Method I
- Design of Singly reinforced sections | Design Method 2
- Solved Numericals for Singly reinforced beam | Method 2
- Moment of Resistance for Singly reinforced sections
- Solved numerical example | Moment of resistance
- Solved numerical example 2 | Guide to singly reinforced sections
Now we will move on with our discussion on “Moment of resistance” and derive the formula for Moment of resistance for balanced section, under-reinforced section and over reinforced section.
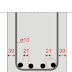
The moment of resistance of the concrete section is the moment of couple formed by the total tensile force (T) in the steel acting at the centre of gravity of reinforcement and the total compressive force (C) in the concrete acting at the centre of gravity (c.g.) of the compressive stress diagram. The moment of resistance is denoted by M.
The distance between the resultant compressive force (C) and tensile force (T) is called the lever arm, and is denoted by z.
From the diagram above, it is clear that the intensity of compressive stress varies from maximum at the top to zero at the neutral axis.
Therefore, centre of gravity of the compressive force is at a distance x/3 from the top edge of the section.
Therefore, z = d-x/3
Moment of resistance is given by,
Mr = C x z
= bx (σcbc/2)(d-x/3)
OR
Mr = T x z
= Ast σst(d – x/3)
For balanced section, the formula is as follows,
Mr = bxc σcbc (d – xc/3)
= Ast σst(d – xc/3)
For under-reinforced section, the formula is as follows,
Mr = T x z
= Ast.σst (d – x/3)
For over-reinforced section, the formula is given as,
Mr = C x z
Mr = bx( σcbc /2) (d – x/3)
Moment of Resistance | Design of Singly reinforced Sections
Moment of Resistance | Guide to design of Singly reinforced Sections
For the design of Singly reinforced Sections article series, we have covered the following:
- Basic definitions and formulas
- Understanding stresses and modular ratios
- Assumptions for singly reinforced sections
- Design procedure for Singly reinforced section – I
- Solved Numericals for Singly reinforced beam | Method I
- Design of Singly reinforced sections | Design Method 2
- Solved Numericals for Singly reinforced beam | Method 2
- Moment of Resistance for Singly reinforced sections
- Solved numerical example | Moment of resistance
- Solved numerical example 2 | Guide to singly reinforced sections
Now we will move on with our discussion on “Moment of resistance” and derive the formula for Moment of resistance for balanced section, under-reinforced section and over reinforced section.
The moment of resistance of the concrete section is the moment of couple formed by the total tensile force (T) in the steel acting at the centre of gravity of reinforcement and the total compressive force (C) in the concrete acting at the centre of gravity (c.g.) of the compressive stress diagram. The moment of resistance is denoted by M.
The distance between the resultant compressive force (C) and tensile force (T) is called the lever arm, and is denoted by z.
From the diagram above, it is clear that the intensity of compressive stress varies from maximum at the top to zero at the neutral axis.
Therefore, centre of gravity of the compressive force is at a distance x/3 from the top edge of the section.
Therefore, z = d-x/3
Moment of resistance is given by,
Mr = C x z
= bx (σcbc/2)(d-x/3)
OR
Mr = T x z
= Ast σst(d – x/3)
The moment of resistance of the concrete section is the moment of couple formed by the total tensile force (T) in the steel acting at the centre of gravity of reinforcement and the total compressive force (C) in the concrete acting at the centre of gravity (c.g.) of the compressive stress diagram. The moment of resistance is denoted by M.
From the diagram above, it is clear that the intensity of compressive stress varies from maximum at the top to zero at the neutral axis.
Therefore, centre of gravity of the compressive force is at a distance x/3 from the top edge of the section.
Therefore, z = d-x/3
Moment of resistance is given by,
Mr = C x z
= bx (σcbc/2)(d-x/3)
OR
Mr = T x z
= Ast σst(d – x/3)






No comments:
Post a Comment
If you are getting more information from civilengineerfriend page please give your comments. Share the page information in your whatsapp group. Subscribe our page to get more information.